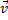
1. A charge q is moving with a uniform velocity . Obtain its Poynting vector  and show that the energy propagates along with the moving charge.

The electric field due to the charge is given by 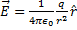. A moving charge can be considered as a current,

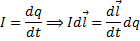
which gives,

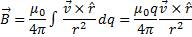
as the integrand does not depend on q.  Thus, the Poynting vector is given by

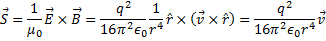
because the radial direction is perpendicular to the direction of velocity.

2. A parallel plate capacitor  with circular plates of radius R is being charged at a uniform rate  by current I in an external circuit.  Obtain an expression for the Poynting vector when it is being charged. Calculate the flow of energy through imaginary cylindrical surface defined by the two plates of the capacitor.

The magnetic field was calculated in the last lecture in terms of the displacement current, it is given by

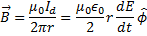
The electric field is directed from the positive plate to the negative plate (defined to be z direction) and is written as 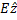. The Poynting vector is given by

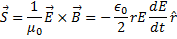
The  rate of energy flow through the imaginary cylindrical surface is obtained by integrating the Poynting vector and is given by (since the surface points inward)

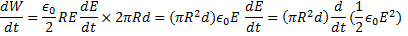
the last relation shows that the energy flow is at the cost of the energy stored in the electric field.